CryptoDB
Multi-party PSM, Revisited: Improved Communication and Unbalanced Communication
| Authors: | |
|---|---|
| Download: | |
| Presentation: | Slides |
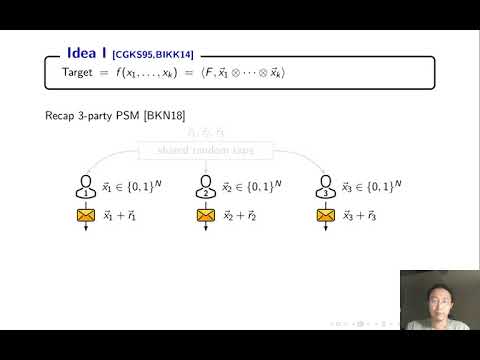
| Abstract: | We improve the communication complexity in the Private Simultaneous Messages (PSM) model, which is a minimal model of non-interactive information-theoretic multi-party computation. The state-of-the-art PSM protocols were recently constructed by Beimel, Kushilevitz and Nissim (EUROCRYPT 2018). We present new constructions of $k$-party PSM protocols. The new protocols match the previous upper bounds when $k=2$ or $3$ and improve the upper bounds for larger $k$. We also construct $2$-party PSM protocols with unbalanced communication complexity. More concretely, - For infinitely many $k$ (including all $k \leq 20$), we construct $k$-party PSM protocols for arbitrary functionality $f:[N]^k\to\{0,1\}$, whose communication complexity is $O_k(N^{\frac{k-1}{2}})$. This improves the former best known upper bounds of $O_k(N^{\frac{k}{2}})$ for $k\geq 6$, $O(N^{7/3})$ for $k=5$, and $O(N^{5/3})$ for $k=4$. - For all rational $0<\eta<1$ whose denominator is $\leq 20$, we construct 2-party PSM protocols for arbitrary functionality $f:[N]\times[N]\to\{0,1\}$, whose communication complexity is $O(N^\eta)$ for one party, $O(N^{1-\eta})$ for the other. Previously the only known unbalanced 2-party PSM has communication complexity $O(\log(N)), O(N)$. |
Video from TCC 2021
BibTeX
@article{tcc-2021-31565,
title={Multi-party PSM, Revisited: Improved Communication and Unbalanced Communication},
booktitle={Theory of Cryptography;19th International Conference},
publisher={Springer},
doi={10.1007/978-3-030-90453-1_7},
author={Léonard Assouline and Tianren Liu},
year=2021
}