CryptoDB
Limits of Polynomial Packings for $\mathbb{Z}_{p^k}$ and $\mathbb{F}_{p^k}$
| Authors: |
|
|---|---|
| Download: | |
| Presentation: | Slides |
| Conference: | EUROCRYPT 2022 |
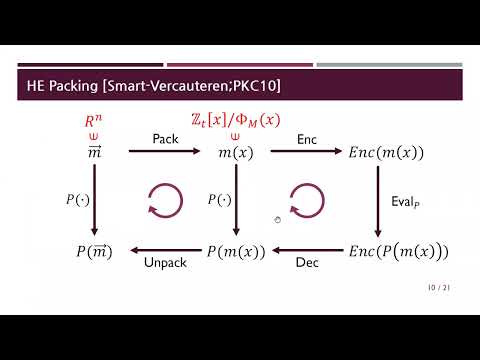
| Abstract: | We formally define polynomial packing methods and initiate a unified study of related concepts in various contexts of cryptography. This includes homomorphic encryption (HE) packing and reverse multiplication-friendly embedding (RMFE) in information-theoretically secure multi-party computation (MPC). We prove several upper bounds and impossibility results on packing methods for $\mathbb{Z}_{p^k}$ or $\mathbb{F}_{p^k}$-messages into $\mathbb{Z}_{p^t}[x]/f(x)$ in terms of (i) packing density, (ii) level-consistency, and (iii) surjectivity. These results have implications on recent development of HE-based MPC over $\mathbb{Z}_{2^k}$ secure against actively corrupted majority and provide new proofs for upper bounds on RMFE. |
Video from EUROCRYPT 2022
BibTeX
@inproceedings{eurocrypt-2022-31889,
title={Limits of Polynomial Packings for $\mathbb{Z}_{p^k}$ and $\mathbb{F}_{p^k}$},
publisher={Springer-Verlag},
author={Jung Hee Cheon and Keewoo Lee},
year=2022
}